Activity 24
MATH 216: Statistical Thinking
Activity 1: Quiz
Given the following sample data: \(\bar{x} = 80\), \(s = 6\), \(n = 25\), and \(\mu_0 = 75\), calculate the test statistic (\(z\)) for a one-tailed test where \(H_a: \mu > 75\).
Use the formula:
\[ z = \frac{\bar{x} - \mu_0}{s/\sqrt{n}} \]
Discuss: After calculating your answer, discuss with a neighbor how you arrived at your result.
- What is the critical value for \(\alpha = 0.05\)?
- Would you reject the null hypothesis based on your calculated \(z\)-score?
Activity 2: Case Studies and Problem-Solving
- Case Study: A nutritionist claims that a new diet reduces the average calorie intake below 2200 calories/day. The sample data shows \(\bar{x} = 2150\), \(s = 120\), \(n = 35\).
- Task: Conduct a hypothesis test to determine if the data supports the nutritionist’s claim at \(\alpha = 0.05\).
- Analysis: Calculate the test statistic (\(z\)) and p-value. Determine whether to reject the null hypothesis.
- Discussion: Discuss the implications of your findings. What are the potential consequences of a Type I or Type II error in this context?
Activity 3: Group Activities with Real Data
An analyst claims that a new billing system changes the average monthly bill from $100.
- Sample Data: \(\bar{x} = 105\), \(s = 10\), \(n = 40\)
- Test: \(H_0: \mu = 100\) vs. \(H_a: \mu \neq 100\)
- Calculation: \(z = \frac{105 - 100}{10/\sqrt{40}} \approx 3.16\)
- Conclusion: Reject \(H_0\) if \(|z| > 1.96\) (at \(\alpha = 0.05\)).
- Based on the test statistic \(z = 2.53\), calculate the p-value and determine whether to reject the null hypothesis at a significance level of \(\alpha = 0.05\). Does the p-value suggest strong evidence against the null hypothesis?
- Construct the 95% confidence interval for the population mean using the sample data (\(\bar{x} = 105\), \(s = 10\), \(n = 40\)). Based on the confidence interval, would you reject the null hypothesis that the population mean is \(100\)?
- Based on the confidence interval, determine whether you would reject the null hypothesis that the population mean is \(100\).
Activity 4
The sample of 100 drug-injected rats yielded the results (in seconds) shown in the following Table. Conduct a hypothesis test to determine if the mean reaction time differs from 1.2 seconds at \(\alpha = 0.01\). Assume \(\bar{x} = 1.05\), \(s = 0.49\), \(n = 100\)
\[\begin{align*} H_0: \mu=1.2 \\ H_a: \mu \neq 1.2 \end{align*}\]
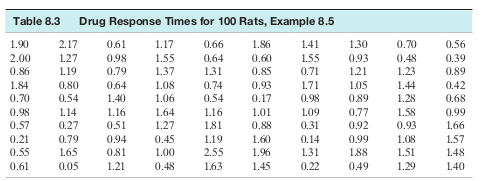
- What is the calculated \(z\)-score?
- What is the p-value, and does it support rejecting the null hypothesis?